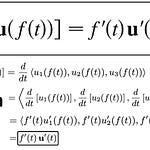YouTube - Summary - Notes - Playlist
In this video I solve the quadratic formula again, but this time using the PQ method instead of the complete the squares method that I used earlier. This is because the PQ method is utilized throughout the cubic formula proof, so this is a good preview. The procedure starts off the same as before, by dividing out the quadratic equation by a, but now we set p = b/a and q = c/a. We can now shift the parabola, which is just a quadratic equation, by setting x = y + k in order to cancel out the px term. Expanding out, we find that the value k = -p/2 cancels out the px term, thus allowing us to solve for y. Plugging y back into x, we obtain the PQ version of the quadratic formula.
Timestamps:
Solve quadratic formula using PQ substitution: 0:00
Set p = b/a and q = c/a to get PQ version of quadratic equation: 0:40
Shift parabola to remove px term by setting x = y + k: 1:02
Set k = -p/2 to cancel out px terms: 2:33
Rearrange to solve for y: 5:13
Plug y back into x to obtain PQ formula: 5:37
PQ formula: 6:30