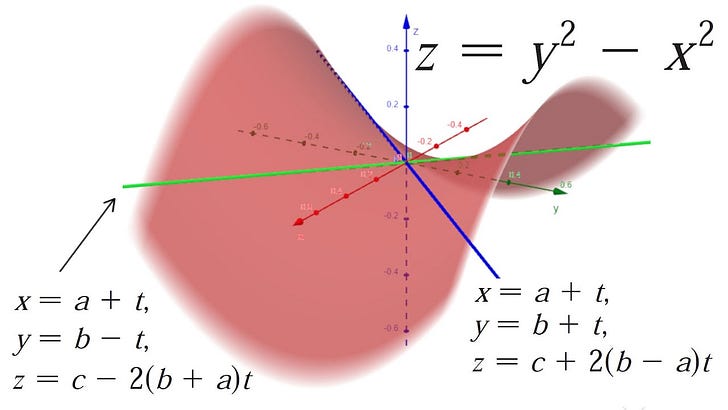
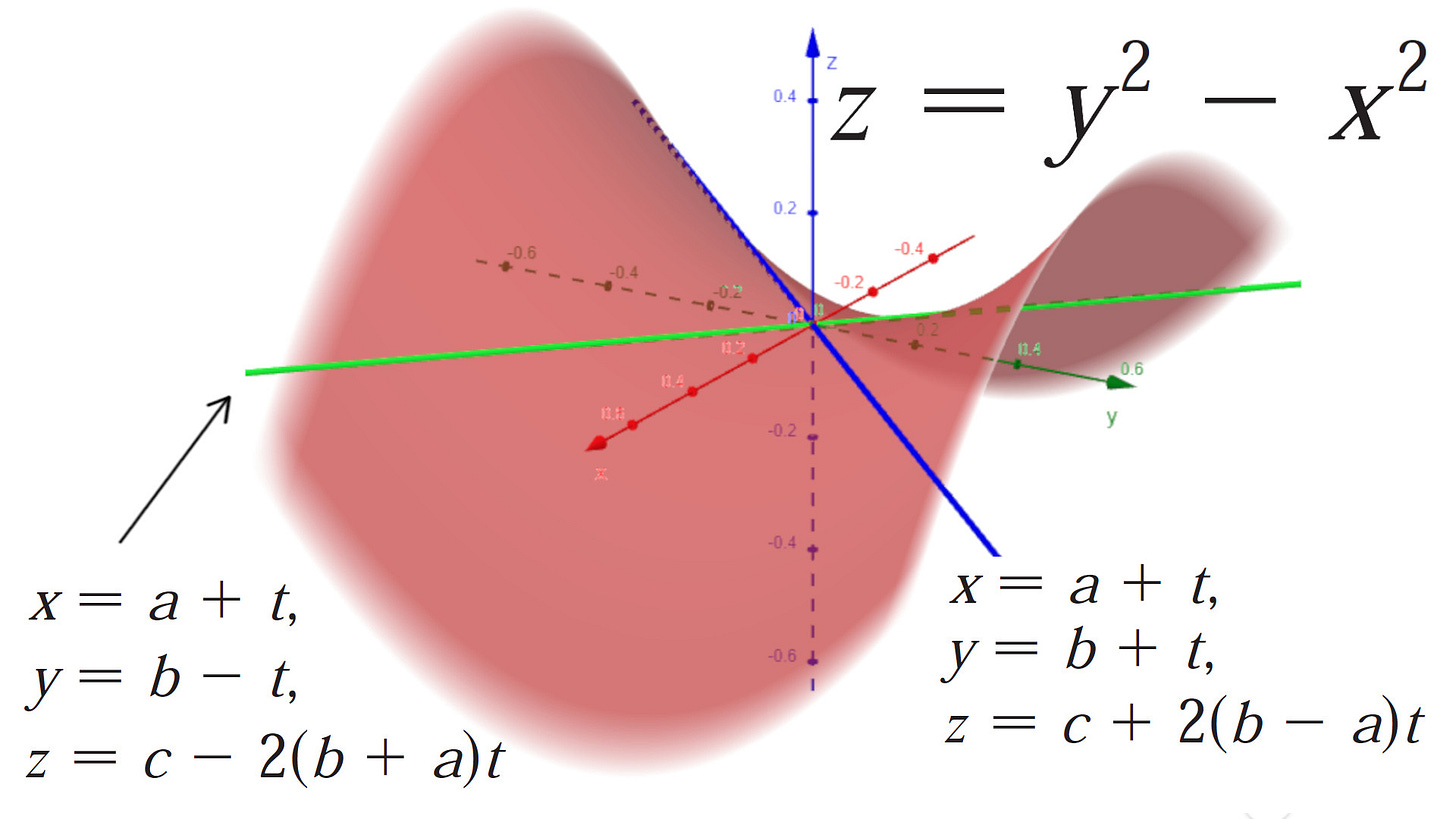
A Hyperbolic Paraboloid Can Be Generated by Straight lines

Only other quadric surfaces that can be generated by lines are: cylinders, cones, and hyperboloids of one sheet.

Full video - Video notes - Video sections playlist - Infinite Sequences and Series playlist

In this video I show that a hyperbolic paraboloid can be generated entirely by two sets of lines, thus classifying it as a Ruled Surface. The only quadric surfaces that are ruled surfaces are cylinders, cones, and hyperboloids of one sheet. All of these can be generated by the motion of straight lines. A hyperbolic paraboloid has horizontal traces that are hyperbolas and vertical traces that are parabolas. I show that two sets of lines in parametric equations can be plugged directly into the equation of the hyperboloid paraboloid and obtain a point on it. I also play around with the lines using GeoGebra's 3D graphing calculator to show that the lines are always on the hyperbolic paraboloid, which is pretty amazing when you think about it!

• GeoGebra 3D graphing calculator: https://www.geogebra.org/3d/tabys4ec